Chapter 6 Review Test 4th Grade Answer Key

Improve your subject noesis and problem-solving skills with the help of Become Math Course 4 Respond Central Homework FL Chapter 6 Fraction Equivalence and Comparing Review/Test Pdf. All the questions and answers covered in this HMH Go Math Course 4 Review/Examination Respond Key are prepared by math experts and back up students to score good marks in the exam.

Become Math Class iv Answer Key Homework FL Chapter 6 Fraction Equivalence and Comparison Review/Exam

Students who are searching for the all-time review/test guide can refer to this helpful Get Math Grade 4 Solution Primal Homework FL Chapter 6 Fraction Equivalence and Comparing Review/Test . As information technology includes all the concepts in Affiliate 6 Fraction Equivalence and Comparing. So, students tin discover the methods to solve the problems easily and tin explore the knowledge by giving the question from Review/Exam. Click on the respective link and download it for better practice.

Affiliate vi: Review/Test

• Review/Test – Page No. 261
• Review/Test – Page No. 262
• Review/Examination – Page No. 263
• Review/Examination – Page No. 264

Review/Test – Page No. 261

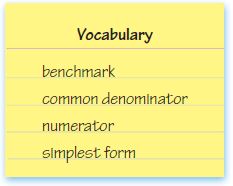
Choose the best term from the box.

Question 1.
A ________________ is a common multiple of 2 or more denominators.
________

Answer:
A common denominator is a common multiple of two or more denominators..

Question 2.
A fraction is in _________________ when the numerator and denominator take only 1 every bit a mutual factor
________

Reply:
A fraction is in simplest form when the numerator and denominator take only one every bit a common gene.

Question three.
A ________________ is a known size or amount that helps yous understand another size or amount.
________

Respond:
A criterion is a known size or amount that helps you empathize another size or amount.

Write 2 equivalent fractions.

Question iv.
\(\frac{4}{6}\)

Respond: \(\frac{6}{9}\) and \(\frac{viii}{12}\).

Caption:
To notice equivalent fractions we volition multiply its numerator and denominator by the same number. Firstly we volition calculate GCF for the given fraction i.e \(\frac{4}{6}\), the GCF for (4,half dozen) is two. Equally GCF is not equal to 1, we will divide the numerator and denominator by 2. By dividing with ii we will get the fraction equally \(\frac{2}{3}\). Now we will multiply the numerator and denominator with iii,
So the fraction will be 3(\(\frac{two}{3}\))
= \(\frac{6}{9}\). For the 2d equivalent fraction, we will multiply numerator and denominator with 4,
And then the fraction will be four(\(\frac{two}{three}\))
= \(\frac{eight}{12}\).
Then, the two equivalent fractions of \(\frac{4}{6}\) are \(\frac{6}{ix}\) and \(\frac{viii}{12}\).

Question 5.
\(\frac{vi}{10}\)

Answer: \(\frac{9}{15}\) and \(\frac{12}{20}\).

Explanation:
To find equivalent fractions nosotros will multiply its numerator and denominator by the same number. Firstly nosotros will summate GCF for the given fraction i.due east \(\frac{half dozen}{10}\), the GCF for (6,10) is 2. As GCF is non equal to 1, we will divide the numerator and denominator past two. By dividing with 2 we volition get the fraction as \(\frac{3}{5}\). Now we will multiply the numerator and denominator with 3,
And then the fraction will be 3(\(\frac{3}{5}\))
= \(\frac{nine}{15}\). For the 2nd equivalent fraction, we will multiply numerator and denominator with 4,
So the fraction volition exist iv(\(\frac{3}{5}\))
= \(\frac{12}{20}\).
Then, the ii equivalent fractions of \(\frac{3}{v}\) are \(\frac{ix}{15}\) and \(\frac{12}{twenty}\).

Question 6.
\(\frac{ii}{8}\)

Respond: \(\frac{3}{12}\) and \(\frac{4}{16}\).

Explanation:
To observe equivalent fractions we will multiply its numerator and denominator by the aforementioned number. Firstly nosotros will calculate GCF for the given fraction i.e \(\frac{2}{8}\), the GCF for (ii,viii) is 2. Equally GCF is non equal to one, nosotros will divide the numerator and denominator by ii. Past dividing with 2 we will become the fraction as \(\frac{1}{4}\). Now we volition multiply the numerator and denominator with 3,
And so the fraction will be 3(\(\frac{i}{4}\))
= \(\frac{three}{12}\). For the 2d equivalent fraction, we will multiply numerator and denominator with 4,
So the fraction will be iv(\(\frac{ane}{4}\))
= \(\frac{4}{16}\).
And then, the two equivalent fractions of \(\frac{2}{8}\) are \(\frac{three}{12}\) and \(\frac{4}{16}\).

Write each pair of fractions as a pair of fractions with a common denominator.

Question seven.
\(\frac{3}{4} \text { and } \frac{7}{viii}\)

Reply: \(\frac{6}{8}\) , \(\frac{7}{viii}\).

Explanation:
To get the mutual denominators we will multiply \(\frac{iii}{four}\) with 2, so that the fraction will be \(\frac{6}{viii}\). As the other fraction is \(\frac{7}{8}\). Then the denominators are the same.

Question 8.
\(\frac{2}{3} \text { and } \frac{1}{4}\)

Answer: \(\frac{viii}{12}\) and \(\frac{iii}{12}\).

Explanation:
To get the common denominators we will multiply \(\frac{2}{iii}\) with iv and \(\frac{one}{4}\) with three, so that the fractions will exist \(\frac{8}{12}\) and \(\frac{iii}{12}\). So the denominators are same.

Question 9.
\(\frac{vii}{ten} \text { and } \frac{four}{5}\)

Answer: \(\frac{7}{ten}\) and \(\frac{8}{10}\).

Explanation:
To get the common denominators we will multiply \(\frac{4}{v}\) with 2, so that the fraction will be \(\frac{8}{10}\). As the other fraction is \(\frac{vii}{10}\). And the denominators are same.

Compare. Write <, >, or five.

Question x.
\(\frac{five}{8}\) _____ \(\frac{5}{12}\)

Answer: \(\frac{v}{8}\) > \(\frac{5}{12}\).

Explanation:
To compare \(\frac{five}{8}\) and \(\frac{v}{12}\) starting time we will find LCM of 8 and 12.
And the LCM of (8,12) is 24. Now nosotros will multiply \(\frac{5}{8}\) with 3 and \(\frac{five}{12}\) with 2, then the fraction volition exist \(\frac{15}{24}\) and the other fraction is \(\frac{ten}{24}\).
So \(\frac{15}{24}\) is greater than \(\frac{ten}{24}\).

Question 11.
\(\frac{x}{12}\) _____ \(\frac{5}{6}\)

Answer: \(\frac{10}{12}\) = \(\frac{5}{six}\).

Explanation:
To compare \(\frac{10}{12}\) and \(\frac{5}{6}\),first we will discover LCM of 12 and 6.
And the LCM of (12,6) is 12. Now nosotros will multiply \(\frac{5}{6}\) with 2, so the fraction will be \(\frac{10}{12}\) and the other fraction is \(\frac{x}{12}\).
So \(\frac{ten}{12}\) is equal to \(\frac{10}{12}\).

Question 12.
\(\frac{1}{ii}\) _____ \(\frac{3}{x}\)

Reply: \(\frac{1}{ii}\) > \(\frac{iii}{x}\).

Explanation:
To compare \(\frac{1}{two}\) and \(\frac{3}{10}\) kickoff nosotros volition detect LCM of 2 and 10.
And the LCM of (two,ten) is 10. Now nosotros volition multiply \(\frac{1}{two}\) with 5, then the fraction will be \(\frac{five}{10}\) and the other fraction is \(\frac{iii}{x}\).
So \(\frac{5}{x}\) is greater than \(\frac{3}{10}\).

Question 13.
\(\frac{1}{four}\) _____ \(\frac{ane}{three}\)

Answer: \(\frac{1}{iv}\) < \(\frac{1}{3}\).

Caption:
To compare \(\frac{1}{4}\) and \(\frac{i}{three}\) kickoff we will find LCM of four and 3.
And the LCM of (four,3) is 12. At present nosotros volition multiply \(\frac{1}{4}\) with three and \(\frac{1}{3}\) with 4, so the fraction will be \(\frac{3}{12}\) and the other fraction is \(\frac{4}{12}\).
So \(\frac{3}{12}\) is less than \(\frac{4}{12}\).

Write the fractions in order from least to greatest.

Question 14.
\(\frac{2}{three}, \frac{iii}{4}, \frac{1}{6}\)

Respond: \(\frac{i}{six}\) < \(\frac{2}{3}\)< \(\frac{three}{4}\).

Explanation:
To write the fraction from least to greatest we will find LCM of three,four,6. And the LCM of (3,4,6) is 12. Now nosotros will multiply
\(\frac{2}{three}\) with 4 and \(\frac{three}{4}\) with 3 and \(\frac{1}{6}\) with 2, so the fraction will be
\(\frac{8}{12}\) and \(\frac{9}{12}\), \(\frac{ii}{12}\)
So \(\frac{ii}{12}\) is less than \(\frac{8}{12}\) is less than \(\frac{9}{12}\).

Question fifteen.
\(\frac{7}{ten}, \frac{4}{v}, \frac{1}{2}, \frac{four}{12}\)

Respond: \(\frac{4}{12}\) < \(\frac{ane}{2}\)< \(\frac{7}{10}\)< \(\frac{iv}{5}\).

Explanation:
To write the fraction from to the lowest degree to greatest nosotros will find LCM of x,5,2,12. And the LCM of (10,v,two,12) is 60. Now we will multiply
\(\frac{vii}{x}\) with half-dozen and \(\frac{iv}{5}\) with 12 and \(\frac{1}{2}\) with 30 and \(\frac{4}{12}\) with 5 , and then the fraction will exist
\(\frac{42}{threescore}\) and \(\frac{48}{60}\), \(\frac{xxx}{lx}\), \(\frac{20}{60}\)
Then \(\frac{20}{60}\) is less than \(\frac{30}{60}\) is less than \(\frac{42}{60}\) is less than
\(\frac{48}{60}\).

Review/Test – Page No. 262

Fill in the bubble completely to evidence your answer.

Question 16.
Paco needs at least \(\frac{3}{eight}\) yard of twine to build a model ship. How much twine could he buy?
Options:
a. \(\frac{3}{10}\) yard
b. \(\frac{1}{4}\) yard
c. \(\frac{iii}{5}\) one thousand
d. \(\frac{1}{8}\) yard

Answer: c.

Explanation:

a) iii/10 yard. As we know that for ii rational numbers with the aforementioned numerator but with different denominators the number whose denominator is smaller is a greater quantity.
Hence three/x < 3/eight. And option a is incorrect.

b) 1/four chiliad. Equally to compare to rational numbers we have to either brand the numerator equal or their denominator equal. Hence hither we multiply and dissever 1/4 past 2 to get 8 in the denominator. Every bit 2/viii < 3/viii
since the denominator is the same and the number with the aforementioned denominator but with different numerators are compared as whose numerator is greater is a greater quantity. And the option b is incorrect.

c) 3/4 yard. Every bit both the numbers have the same numerator but different denominator and we know that for two rational numbers with the aforementioned numerator only with dissimilar denominators the number whose denominator is smaller is a greater quantity. Every bit 3/8 < 3/four, then pick c is correct.

d) 1/viii yard. As both the numbers take the aforementioned denominator and we know that for 2 rational numbers with the same denominator but with the dissimilar numerators, the number whose numerator is smaller is a smaller quantity. And so one/8 < 3/eight and the option d is incorrect.

Question 17.
Rachel, Nancy, and Diego were in a angling contest. Rachel'southward fish was \(\frac{7}{8}\) foot long, Nancy'south fish was \(\frac{1}{iv}\) pes long, and Diego'south fish was \(\frac{one}{2}\) human foot long. What are the lengths of the fish in gild from least to greatest?
Options:
a. \(\frac{7}{eight}\) foot, \(\frac{1}{ii}\) foot, \(\frac{ane}{iv}\) foot
b. \(\frac{1}{ii}\) foot, \(\frac{7}{8}\) pes, \(\frac{ane}{iv}\) foot
c. \(\frac{7}{8}\) foot, \(\frac{1}{4}\) foot, \(\frac{1}{2}\) foot
d. \(\frac{1}{4}\) pes, \(\frac{1}{2}\) foot, \(\frac{vii}{viii}\) foot

Reply: d

Caption:
Every bit Rachel'southward fish was \(\frac{vii}{8}\) human foot long, Nancy'south fish was \(\frac{one}{4}\) foot long, Diego's fish was \(\frac{1}{two}\) human foot long, and so to find the lengths of the fish in guild from least to greatest we volition find the LCM of (eight,4,ii), so the LCM of (viii,4,2) is 8 and we will multiply \(\frac{i}{4}\) with two and \(\frac{1}{2}\) with 4, so the fraction will be \(\frac{2}{8}\) and \(\frac{iv}{8}\). The lengths of the fish in order from least to greatest are  \(\frac{ii}{8}\), latex]\frac{four}{viii}[/latex], latex]\frac{seven}{8}[/latex]

Question 18.
Amy needs \(\frac{six}{viii}\) gallon of fruit juice to brand punch. She needs an equal corporeality of sparkling h2o. How much sparkling water does she need?
Options:
a. \(\frac{ii}{8}\) gallon
b. \(\frac{1}{ii}\) gallon
c. \(\frac{ii}{iii}\) gallon
d. \(\frac{3}{4}\) gallon

Answer: d

Caption:
Amy needs \(\frac{6}{8}\) gallon of fruit juice to brand punch and she needs an equal amount of sparkling water, so Amy needs \(\frac{6}{8}\) or \(\frac{3}{4}\) gallon.

Question 19.
Gavin is building a model of a kitchen. In the model, \(\frac{ii}{v}\) of the flooring tiles are white, \(\frac{ane}{2}\) of the floor tiles are yellow, and \(\frac{1}{ten}\) of the floor tiles are dark-brown. How many floor tiles could be in the model?
Options:
a. 2
b. 5
c. 10
d. 17

Answer: c

Caption:
Every bit Gavin is building a model of a kitchen and \(\frac{ii}{5}\) of the floor tiles are white, \(\frac{1}{2}\) of the floor tiles are yellow, and \(\frac{one}{10}\) of the floor tiles are brown. To find the full number of tiles we will add up all color tiles. For that, we will multiply \(\frac{one}{ii}\) with 5 and \(\frac{ii}{v}\) with 2 to fix the denominators equal. Then the fractions will be \(\frac{five}{x}\) and \(\frac{4}{ten}\). Now add all 3
\(\frac{5}{10}\)+\(\frac{4}{10}\)+\(\frac{1}{10}\)
= 10.
And so the number of floor tiles modeled is ten

Review/Test – Folio No. 263

Fill in the chimera completely to show your answer.

Question 20.
Beak has plenty money to purchase no more than \(\frac{1}{2}\) pound of cheese. How much cheese could he purchase?
Options:
a. \(\frac{1}{3}\) pound
b. \(\frac{iv}{vi}\) pound
c. \(\frac{5}{8}\) pound
d. \(\frac{3}{4}\) pound

Answer: a

Caption:
As Pecker has enough money to purchase no more than \(\frac{one}{2}\) pound of cheese, so he needs to buy \(\frac{1}{iii}\) pounds.

Question 21.
Students planted 6 equal-size gardens on Earth Day. They divided each garden into 3 equal sections and planted herbs in 2 of the three sections. What fraction of the gardens did the students plant with herbs?
Options:
a. \(\frac{three}{vi}\)
b. \(\frac{2}{6}\)
c. \(\frac{6}{eighteen}\)
d. \(\frac{12}{xviii}\)

Reply: d

Explanation:
Equally students planted half-dozen equal-size gardens on Earth Mean solar day, and they divided each garden into 3 equal sections and planted herbs in two of the 3 sections, so the fraction of the gardens did the students plant with herbs are we demand to multiply 6×iii and will get 18 sections in all gardens, then we need to multiply 2×six and get 12 sections are herbs. So, 12 out of 18 are herbs i.e \(\frac{12}{18}\).

Question 22.
Noah and Leslie live the same altitude from school. Which could be the distances they live from school?
Options:
a. \(\frac{vii}{100}\) kilometer and \(\frac{7}{ten}\) kilometer
b. \(\frac{five}{10}\) kilometer and \(\frac{1}{five}\) kilometer
c. \(\frac{80}{100}\) kilometer and \(\frac{8}{10}\) kilometer
d. \(\frac{six}{10}\) kilometer and \(\frac{2}{five}\) kilometer

Answer: c.

Explanation:
The option c is correct, as \(\frac{80}{100}\) km is equal to \(\frac{8}{ten}\) when it is reduced.

Question 23.
Keiko needs \(\frac{8}{12}\) yard of fabric to end her quilt. What is \(\frac{8}{12}\) written in simplest form?
Options:
a. \(\frac{4}{6}\)
b. \(\frac{2}{iii}\)
c. \(\frac{3}{4}\)
d. \(\frac{one}{two}\)

Reply: b

Explanation:
Equally Keiko needs \(\frac{8}{12}\) yard of cloth to stop her quilt and the simplest course of \(\frac{viii}{12}\) is \(\frac{ii}{3}\).

Review/Examination – Page No. 264

Question 24.
Sam needs \(\frac{four}{6}\) cup of laundry detergent for his laundry. The cap on top of the laundry detergent holds \(\frac{1}{iii}\) loving cup. He has i capful of detergent. Does he have enough? Explain.

Answer: Sam does non have enough.

Explanation:
As Sam needs \(\frac{4}{6}\) cup of laundry detergent for his laundry and the cap holds just \(\frac{ane}{three}\) and Sam has 1 capful of detergent, and Sam needs 2 cups instead of ane cup because \(\frac{4}{6}\) is equivalent to \(\frac{2}{iii}\) and Sam only has \(\frac{1}{3}\) cup, so he needs 2 cups.

Question 25.
The table shows the distances of some places in town from the school.

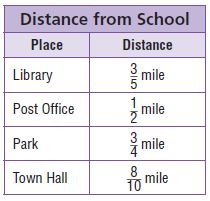
A. Are any of the places shown in the table closer than \(\frac{i}{2}\) mile to school? Explain how yous know.

Answer: Library \(\frac{3}{v}\) mile.

Explanation:
To find which place is closer, we will detect the LCM of the denominators i.e (5,two,4,10). And the LCM of (5,2,iv,10) is 20, and then we will divide \(\frac{3}{5}\) with 4, \(\frac{1}{2}\) with 10, \(\frac{3}{four}\) with 5 and \(\frac{8}{10}\) with 2. So that the fractions will take same denominators and nosotros tin notice hands which place is closer. And the fractions after multiplying are \(\frac{12}{20}\), \(\frac{10}{20}\), \(\frac{15}{twenty}\) and \(\frac{16}{20}\). Then the places closer than \(\frac{1}{ii}\) mile to schoolhouse are post office which is \(\frac{x}{20}\) mile and next place is library which is \(\frac{12}{20}\) mile.

Question 25.
B. Are any of the places shown in the table the same altitude from schoolhouse? Explain how y'all know.

Answer: Yes.

Question 25.
C. Which identify is farthest from school? Explicate.

Answer: Townhall.

Explanation:
Townhall is the farthest from the schoolhouse every bit it's distance is \(\frac{8}{10}\) mile.

Decision:

Ace up your preparation with this Go Math Grade four Reply Key Chapter 6 Homework FL in pdf download. Hence, you can exercise finer and score practiced grades in the examination. Here, we take curated a detailed explanation of questions from ch six and provided Get Math Form four Answer Key Homework FL Chapter 6 Fraction Equivalence and Comparing Review/Test. So, We suggest students to understand the topics and apply them in the existent globe.

jacobsennoeve1936.blogspot.com

Source: https://ccssmathanswers.com/go-math-grade-4-answer-key-homework-fl-chapter-6-fraction-equivalence-and-comparison-review-test/

Share this post